
 Perhaps
as a result of having grown up with the movie, "Gypsy Moths", many skydivers of
the "baby boomer" generation engaged in flights of fancy that had their origins
in barnstorming days. In 1977, skydiver Pat Quiqley remarked , "someday people
will be landing on runways with jumpsuits". His quip was an immediate response
to a creative fellow who had shown up at the Fulton Missouri dropzone, looking
like Chewbaka, ready to jump wearing huge furry pajamas.
We watched him wookie through 1000 feet struggling to find his ripcord
in the confused folds of fabric. After a long walk, and probably some soul
searching he returned to the hanger with a sheepish look on his face, escorted
by aviation pioneers of the past who had decided to let him live another day.
Jay Weaver, the dropzone owner, rendered the obligatory safety lecture, while
the rest of us rendered expressions ranging from looks of unbelief to rolling
eyes. We all knew there was something to this concept, we just couldn't
figure out how to do it. At the time, the Nelson brothers had a mascot that
featured special little wings. The notion stuck with me to the point that
when no one was looking, I built a pair and tested them by jumping on my
trampoline... At this point it became clear that there were two portions to this
fantasy, the recreational air portion, similar to skysurfing, and the stunt portion
that wondered, "could you land this?".
Perhaps
as a result of having grown up with the movie, "Gypsy Moths", many skydivers of
the "baby boomer" generation engaged in flights of fancy that had their origins
in barnstorming days. In 1977, skydiver Pat Quiqley remarked , "someday people
will be landing on runways with jumpsuits". His quip was an immediate response
to a creative fellow who had shown up at the Fulton Missouri dropzone, looking
like Chewbaka, ready to jump wearing huge furry pajamas.
We watched him wookie through 1000 feet struggling to find his ripcord
in the confused folds of fabric. After a long walk, and probably some soul
searching he returned to the hanger with a sheepish look on his face, escorted
by aviation pioneers of the past who had decided to let him live another day.
Jay Weaver, the dropzone owner, rendered the obligatory safety lecture, while
the rest of us rendered expressions ranging from looks of unbelief to rolling
eyes. We all knew there was something to this concept, we just couldn't
figure out how to do it. At the time, the Nelson brothers had a mascot that
featured special little wings. The notion stuck with me to the point that
when no one was looking, I built a pair and tested them by jumping on my
trampoline... At this point it became clear that there were two portions to this
fantasy, the recreational air portion, similar to skysurfing, and the stunt portion
that wondered, "could you land this?".

At the 1993 Parachute Industry Association conference, I broached the idea of non-parachute assisted minimalist gliding to John LeBlanc, a parachute designer at Performance Designs in Florida and Tony Domenico, owner of Square-One parachute lofts in Southern California.

John LeBlanc's suggestion was to create some kind of a jumpsuit with inflatable ram-air wings. We had all seen the Krueger balloon suit slow people down to 85 mph or so, but it was a pure drag affair inflating the occupant to Michelin man freefall proportions. John wanted to build cells into the wing area under the arm. I wondered if someone could get good enough on rollerblades to land at high speeds.

At the same PIA convention,Tony Domenico suggested some kind of rigid manta-ray like shape as we sketched concepts over chicken salad in a Florida hotel.

There was something beautiful about his suggestion to look at creatures other than birds from the animal kingdom. Manta's were especially inviting, their wing spans can exceed twenty feet.
 Struggling
with everything from condor's to cruise missles I had been imagining telescoping
and "pop-out" wing designs, even an expanding oriental fan idea.
Struggling
with everything from condor's to cruise missles I had been imagining telescoping
and "pop-out" wing designs, even an expanding oriental fan idea. 
Angus Rupert had even suggested some kind of deployable rotor. In my mind I added a winglet to his idea to make it self starting. However the contrast between parachutes and a descending "pair of scissors" was too much and I abandoned that concept. The notion of an activating winglet was to remain.
 I
mentioned this to the distinguished aviation designer, Roy Haggard in 1995.
Roy had been a player in some important aviation firsts including Jim Handbury's
triple person hang-glider flight off half dome, and Dar Robinson's jump across
the grand canyon, all of which used parachutes for the landing.
Roy also wants to see a successful repeat of the ill-fated Snake River Canyon
jump, and is one of the few people with the background and facilities to pull
it off.
I
mentioned this to the distinguished aviation designer, Roy Haggard in 1995.
Roy had been a player in some important aviation firsts including Jim Handbury's
triple person hang-glider flight off half dome, and Dar Robinson's jump across
the grand canyon, all of which used parachutes for the landing.
Roy also wants to see a successful repeat of the ill-fated Snake River Canyon
jump, and is one of the few people with the background and facilities to pull
it off.
Analysis
If we assume that a stable vehicle can be constructed, and that altitude can be bled off in a manageable way, then the most important aspect of any gliding descent is the minimum velocity that can be attained. The risk of injury in a crash is directly proportional to the kinetic energy at impact. Kinetic energy is expressed as:

so the principal goal should be to make the landing as slow as possible.
Further, at touchdown, it must be the case that the vertical component
of velocity be zero. This is only possible if the lifting body or
device is flying above stall speed, or is in a controlled interaction with
ground effect. For the sake of this elementary analysis, I will assume
that the single factor most controlling landing velocity is
wing loading. Therefore we will proceed to look at the effect
of wing loading in detail. But first, a quick trip to the zoo...
 Several
species of animals fly including squirrels, lizards and even snakes. Flying
squirrels exploit special adaptations for tree to tree descent. These adaptations
include skin that runs from wrist to ankle on both sides. The tail acts
as a stabilizer, airbrake, and rudder. A specialized use of the tail is
seen in South American snakes that engage in tree to tree flights, flying their
bodies as serpentine lifting vehicles while engaging in slithering behaviors that
provide stabilization. Lizards with winged body appendages and webbed feet
of large relative proportion engage in gliding using their four feet as lifting
and control surfaces.
Several
species of animals fly including squirrels, lizards and even snakes. Flying
squirrels exploit special adaptations for tree to tree descent. These adaptations
include skin that runs from wrist to ankle on both sides. The tail acts
as a stabilizer, airbrake, and rudder. A specialized use of the tail is
seen in South American snakes that engage in tree to tree flights, flying their
bodies as serpentine lifting vehicles while engaging in slithering behaviors that
provide stabilization. Lizards with winged body appendages and webbed feet
of large relative proportion engage in gliding using their four feet as lifting
and control surfaces.
Consider the flying squirrel, illustrated above. The wing loading for the squirrel in units of mass per unit area is:
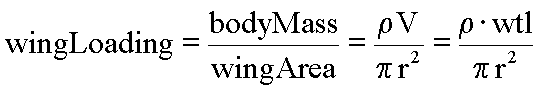
We will assume that this squirrel flies on the aerodynamic equivalent
of:
1) a lifting disk whose radius r is 10 cm.
2) payload body width w of 5 cm.
3) body thickness t of 2 cm.
4) body length l of 20 cm.
We will assume that the density of the squirrel is the same as water,
1 gram per cubic centimeter. Grinding this out we get:
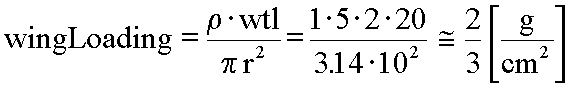
Neglecting the contribution of the tail, this works out to a wing loading of approximately 2/3 gram per square centimeter. Now we want to repeat the calculation for a "man-sized" squirrel which somehow reminds me of a wookie...
We parameterize our squirrel as follows:
1) Consider a lifting disk whose diameter is 2 r.
2) With a payload body width w = r / 2.
3) With a body thickness t = r / 5.
4) With a body length l = 2 r.
Substituting our wookie parameters into the wing loading formula yields:
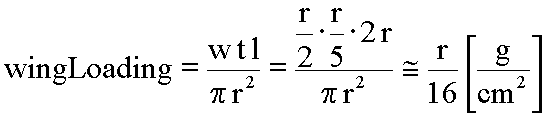
This formula, which I shall call, "The Wookie Formula", is actually quite remarkable.
It states that the "Flying Squirrel Thing" is done at a wing loading that increases linearly with animal size. It is not a constant. In ordinary terms it means, "The bigger you are, the harder you fall".
Now let's apply this to a real person six feet tall. Their radius would be 91 cm. Their "Flying Wookie" wing loading would therefore be 5.72 g/cm2, nine times the wing loading of a squirrel!. To do the "Flying Wookie" a human would have to carry nine times the surface area, or about 254 square feet. The 254 square foot area portends the the use of hang gliders and parachutes; Sports which exist and land at low speeds. Put another way, for a jumpsuit approach to work at flying squirrel speeds, the weight of a flying wookie would have to be 20 pounds, past the capability of even the most avid dieter...
 Because
dynamic pressure grows as the square of velocity, one could fly a vehicle
with only 254/9 = 28 square feet of area if one was willing to increase flight
speed by the square root of nine, or a factor of three (times faster).
For typical figures this works out to about 65 mph, pretty fast for rollerblades!
Pretty fast for a prone landing, but probably within reach of a clever design...
Because
dynamic pressure grows as the square of velocity, one could fly a vehicle
with only 254/9 = 28 square feet of area if one was willing to increase flight
speed by the square root of nine, or a factor of three (times faster).
For typical figures this works out to about 65 mph, pretty fast for rollerblades!
Pretty fast for a prone landing, but probably within reach of a clever design...